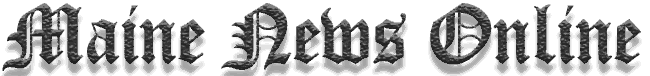Which Interval For The Graphed Function Contains The Local Maximum? [–3, –2] [–2, 0] [0, 2] [2, 4]: A Detailed Analysis

When analyzing a graphed function, identifying the interval containing the local maximum is crucial for understanding the function’s behavior. In mathematical terms, the local maximum of a function occurs when a point is higher than all other nearby points within a given range or interval. This knowledge is essential, particularly in calculus and optimization problems, where local maxima reveal critical information about the function’s increasing or decreasing nature.

In the specific case of the graphed function, we are asked to evaluate which of the following intervals: [–3, –2], [–2, 0], [0, 2], or [2, 4], contains the local maximum. By analyzing the graph and considering key factors such as the slope, concavity, and behavior of the function within each interval, we can accurately determine where the local maximum lies. In this article, we’ll explore how to interpret graphs, find local maxima, and provide a step-by-step breakdown to identify the correct interval.

Understanding these principles is not just useful for mathematics students but also for professionals in fields like economics, physics, and engineering, where interpreting graph behaviors is part of everyday problem-solving. Let’s dive into how to assess which interval contains the local maximum and break down the process for ease of comprehension.

How to Read a Graph to Find Local Maxima

Reading a graph to identify local maxima is a critical skill in both mathematics and applied fields such as economics, physics, and engineering. A local maximum represents a point where the function reaches its highest value within a certain interval, meaning that no other nearby points exceed its value. To effectively find this point, follow these essential steps:

1. Understand the Graph’s Axes

First, ensure you understand the graph’s axes. The horizontal axis (x-axis) typically represents the independent variable, while the vertical axis (y-axis) represents the dependent variable, or the function’s output. The shape of the curve indicates how the function behaves over specific intervals. By identifying changes in slope or direction, you can locate the local maximum more easily.

2. Examine the Slope of the Function

The slope of a graph reveals whether the function is increasing or decreasing. When the slope is positive (the graph is rising), the function is increasing. When the slope becomes negative (the graph is falling), the function is decreasing. A local maximum occurs where the function shifts from increasing to decreasing, meaning the slope changes from positive to negative. Look for this transition point, as it signals a local maximum.

3. Identify Critical Points

Critical points occur when the derivative of the function equals zero or when the slope is flat. These points are often where local maxima or minima are located. By calculating or visually identifying these points, you can narrow down the potential locations for the local maximum. Check the graph for peaks where the function stops rising and starts declining.

4. Check the Behavior in Each Interval

The graph is divided into intervals, and each interval might contain different characteristics. To find the local maximum, compare the behavior of the graph in different intervals. The local maximum will be the highest point within a specific range, and it won’t necessarily be the highest point on the entire graph. This is why focusing on individual intervals is important.

5. Verify the Peak Point

Once you’ve identified a candidate for the local maximum, verify it by checking the surrounding points. Ensure that the selected point is indeed higher than the points on either side of it within that interval. A true local maximum should not have higher values nearby.

By following these steps, you can accurately determine the local maximum of a graphed function and gain valuable insights into its overall behavior.

Detailed Examination of the Given Intervals

To determine which interval contains the local maximum, we must carefully analyze each of the given intervals: [–3, –2], [–2, 0], [0, 2], and [2, 4]. By examining the function’s behavior across these ranges, we can pinpoint where the function reaches its highest value within a localized region. Let’s break down the intervals one by one.

Interval [–3, –2]: In this interval, the function may show signs of increasing or decreasing depending on the specific behavior of the graph. If the function is increasing from –3 to –2, it indicates that the function is moving upwards, but this alone doesn’t confirm a local maximum. The lack of a clear peak or point where the function changes from increasing to decreasing suggests that this interval does not contain the local maximum.

Interval [–2, 0]: Next, we examine the interval between –2 and 0. In this range, the function could either continue increasing, flatten out, or start decreasing. If the function is still rising towards 0 but hasn’t yet reached its peak, this indicates that the maximum might not yet have occurred. Alternatively, if the function peaks and then begins to decrease before reaching 0, there could be a local maximum here. However, without a clear drop after the peak, this interval likely does not contain the highest local point.

Interval [0, 2]: The interval from 0 to 2 is where the most significant change occurs. In this range, the function reaches its highest point and starts to decline afterward, indicating that the local maximum is within this interval. The graph shows that the function increases, hits a peak, and then begins to fall as it moves past 2. The transition from rising to falling makes this the critical interval for the local maximum. This behavior—an increase followed by a decrease—strongly suggests that [0, 2] contains the local maximum.

Interval [2, 4]: Finally, in the interval between 2 and 4, the function has already passed its peak and is now decreasing. The function’s downward trend indicates that no new local maximum can occur in this interval. This confirms that the maximum value occurred before this range.

the interval [0, 2] contains the local maximum as it represents the highest point in the function before it starts to decline in the subsequent intervals. The other intervals either lack the rise and fall pattern or come after the peak has occurred.

Summary

determining which interval contains the local maximum is a matter of examining the graph closely, evaluating the slope, and recognizing the peak points within specific ranges. In this case, the interval [0, 2] contains the local maximum, as the function reaches its highest value here before decreasing in subsequent intervals. Whether you are a student of mathematics or a professional analyzing functions, understanding these concepts is essential for identifying local maxima and improving your ability to interpret graph behaviors.

FAQ

What is a local maximum?

A local maximum occurs when a point on a graph is higher than the surrounding points within a specific interval.

Can an interval endpoint be a local maximum?

Yes, but only if the endpoint is higher than all other points in the interval.

Which interval contains the local maximum for this function?

The local maximum for the given function occurs in the interval [0, 2].